Characteristics of Stepping Motors
Static Torque–Displacement Curves
From the discussion in previous article, it should be clear that the shape of the torque–displacement curve, and in particular the peak static torque, will depend on the internal electromagnetic design of the rotor.
In particular the shapes of the rotor and stator teeth, and the disposition of the stator windings (and permanent magnet(s)) all have to be optimised to obtain the maximum static torque. We now turn to a typical static torque–displacement curve, and look at how it determines motor behaviour.
Several aspects will be discussed, including the explanation of basic stepping (which has already been looked at in a qualitative way); the influence of load torque on step position accuracy; the effect of the amplitude of the winding current; and half-step and mini-stepping operation.
For the sake of simplicity, the discussion will be based on the 30o per step 3-phase VR motor introduced earlier, but the conclusions reached apply to any stepping motor.
Typical static torque–displacement curves for a 3-phase 30o per step VR motor are shown in Figure 1. These show the torque that has to be applied to move the rotor away from its aligned position.
Because of the rotor–stator symmetry, the magnitude of the restoring torque when the rotor is displaced by a given angle in one direction is the same as the magnitude of the restoring torque when it is displaced by the same angle in the other direction, but of opposite sign.
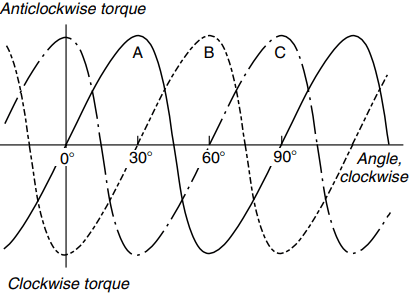
There are three curves in Figure 1, one for each of the three phases, and for each curve we assume that the relevant phase winding carries its full (rated) current. If the current is less than rated, the peak torque will be reduced, and the shape of the curve is likely to be somewhat different.
The convention used in Figure 1 is that a clockwise displacement of the rotor corresponds to a movement to the right, while a positive torque tends to move the rotor anticlockwise.
When only one phase, say A, is energised, the other two phases exert no torque, so their curves can be ignored and we can focus attention on the solid line in Figure 1.
Stable equilibrium positions (for phase A excited) exist at θ = 0o, 90o, 180o and 270o. They are stable (step) positions because any attempt to move the rotor away from them is resisted by a counteracting or restoring torque.
These points correspond to positions where successive rotor poles (which are 90o apart) are aligned with the stator poles of phase A.
There are also four unstable equilibrium positions, (at θ = 45o, 135o, 225o and 315o) at which the torque is also zero. These correspond to rotor positions where the stator poles are midway between two rotor poles, and they are unstable because if the rotor is deflected slightly in either direction, it will be accelerated in the same direction until it reaches the next stable position.
If the rotor is free to turn, it will therefore always settle in one of the four stable positions.
Single-Stepping
If we assume that phase A is energised, and the rotor is at rest in the position θ = 0o (see Figure 1) we know that if we want to step in a clockwise direction, the phases must be energised in the sequence ABCA, etc., so we can now imagine that phase A is switched-off, and phase B is energised instead.
We will also assume that the decay of current in phase A and the build-up in phase B take place very rapidly, before the rotor moves significantly.
The rotor will find itself at θ = 0o, but it will now experience a clockwise torque (see Figure 1) produced by phase B. The rotor will therefore accelerate clockwise, and will continue to experience clockwise torque, until it has turned through 30o. The rotor will be accelerating all the time, and it will therefore overshoot the 30o position, which is of course its target (step) position for phase B.
As soon as it overshoots, however, the torque reverses, and the rotor experiences a braking torque, which brings it to rest before accelerating it back towards the 30o position.
If there was no friction or other cause of damping, the rotor would continue to oscillate; but in practice it comes to rest at its new position quite quickly in much the same way as a damped second-order system.
The next 30o step is achieved in the same way, by switching-off the current in phase B, and switching-on phase C.
In the discussion above, we have recognised that the rotor is acted on sequentially by each of the three separate torque curves shown in Figure 1.
Alternatively, since the three curves have the same shape, we can think of the rotor being influenced by a single torque curve, which ‘jumps’ by one step (30o in this case) each time the current is switched from one phase to the next.
This is often the most convenient way of visualising what is happening in the motor.
Step Position Error and Holding Torque
In the previous discussion the load torque was assumed to be zero, and the rotor was therefore able to come to rest with its poles exactly in line with the excited stator poles. When load torque is present, however, the rotor will not be able to pull fully into alignment, and a ‘step position error’ will be unavoidable.
The origin and extent of the step position error can be appreciated with the aid of the typical torque–displacement curve shown in Figure 2. The true step position is at the origin in the figure, and this is where the rotor would come to rest in the absence of load torque.
If we imagine the rotor is initially at this position, and then consider that a clockwise load (TL) is applied, the rotor will move clockwise, and as it does so it will develop progressively more anticlockwise torque.
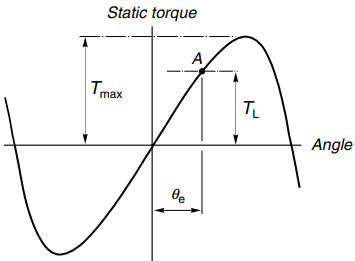
The equilibrium position will be reached when the motor torque is equal and opposite to the load torque, i.e. at point A in Figure 2. The corresponding angular displacement from the step position (θe in Figure 2) is the step position error. The existence of a step position error is one of the drawbacks of the stepping motor.
The motor designer attempts to combat the problem by aiming to produce a steep torque–angle curve around the step position, and the user has to be aware of the problem and choose a motor with a sufficiently steep curve to keep the error within acceptable limits.
In some cases this may mean selecting a motor with a higher peak torque than would otherwise be necessary, simply to obtain a steep enough torque–angle curve around the step position.
As long as the load torque is less than Tmax (see Figure 2), a stable rest position is obtained, but if the load torque exceeds Tmax, the rotor will be unable to hold its step position. Tmax is therefore known as the ‘holding’ torque.
The value of the holding torque immediately conveys an idea of the overall capability of any motor, and it is – after step angle – the most important single parameter, which is looked for in selecting a motor.
Often, the adjective ‘holding’ is dropped altogether: for example ‘a 1-Nm motor’ is understood to be one with a peak static torque (holding torque) of 1 Nm.
Half Stepping
We have already seen how to step the motor in 30o increments by energising the phases one at a time in the sequence ABCA, etc. Although this ‘one-phase-on’ mode is the simplest and most widely used, there are two other modes, which are also frequently employed. These are referred to as the ‘two-phase-on’ mode and the ‘half-stepping’ mode.
The two-phase-on can provide greater holding torque and a much better damped single-step response than the one-phase-on mode; and the half-stepping mode permits the effective step angle to be halved – thereby doubling the resolution – and produces a smoother shaft rotation.
In the two-phase-on mode, two phases are excited simultaneously. When phases A and B are energised, for example, the rotor experiences torques from both phases, and comes to rest at a point midway between the two adjacent full step positions.
If the phases are switched in the sequence AB, BC, CA, AB, etc., the motor will take full (30o) steps, as in the one-phase-on mode, but its equilibrium positions will be interleaved between the full step positions.
To obtain ‘half stepping’ the phases are excited in the sequence A, AB, B, BC, etc., i.e. alternately in the one-phase-on and two-phase-on modes. This is sometimes known as ‘wave’ excitation, and it causes the rotor to advance in steps of 15o, or half the full step angle.
As might be expected, continuous half stepping usually produces a smoother shaft rotation than full stepping, and it also doubles the resolution.
We can see what the static torque curve looks like when two phases are excited by superposition of the individual phase curves. An example is shown in Figure 3, from which it can be seen that for this machine, the holding torque (i.e. the peak static torque) is higher with two phases excited than with only one excited. The stable equilibrium (half-step) position is at 15o, as expected.
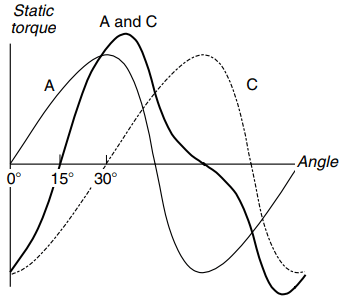
The price to be paid for the increased holding torque is the increased power dissipation in the windings, which is doubled as compared with the one-phase-on mode. The holding torque increases by a factor less than two, so the torque per watt (which is a useful figure of merit) is reduced.
A word of caution is needed in regard to the addition of the two separate one-phase-on torque curves to obtain the two-phase-on curve. Strictly, such a procedure is only valid where the two phases are magnetically independent, or the common parts of the magnetic circuits are unsaturated. This is not the case in most motors, in which the phases share a common magnetic circuit, which operates under highly saturated conditions.
Direct addition of the one-phase-on curves cannot therefore be expected to give an accurate result for the two-phase-on curve, but it is easy to do, and provides a reasonable estimate.
Apart from the higher holding torque in the two-phase-on mode, there is another important difference which distinguishes the static behaviour from that of the one-phase-on mode.
In the one-phase-on mode, the equilibrium or step positions are determined solely by the geometry of the rotor and stator: they are the positions where the rotor and stator are in line.
In the two-phase-on mode, however, the rotor is intended to come to rest at points where the rotor poles are lined-up midway between the stator poles. This position is not sharply defined by the ‘edges’ of opposing poles, as in the one-phase-on case; and the rest position will only be exactly midway if
- there is exact geometrical symmetry and, more importantly
- the two currents are identical.
If one of the phase currents is larger than the other, the rotor will come to rest closer to the phase with the higher current, instead of halfway between the two. The need to balance the currents to obtain precise half stepping is clearly a drawback to this scheme.
Paradoxically, however, the properties of the machine with unequal phase currents can sometimes be turned to good effect, as we now see.
Step Division – Mini-Stepping
There are some applications (e.g. in printing and phototypesetting) where very fine resolution is called for, and a motor with a very small step angle – perhaps only a fraction of a degree – is required.
We have already seen that the step angle can only be made small by increasing the number of rotor teeth and/or the number of phases, but in practice it is inconvenient to have more than four or five phases, and it is difficult to manufacture rotors with more than 50–100 teeth. This means it is rare for motors to have step angles below about 1o.
When a smaller step angle is required a technique known as mini-stepping (or step division) is used. Mini-stepping is a technique based on two-phase-on operation which provides for the subdivision of each full motor step into a number of ‘substeps’ of equal size.
In contrast with half stepping, where the two currents have to be kept equal, the currents are deliberately made unequal.
By correctly choosing and controlling the relative amplitudes of the currents, the rotor equilibrium position can be made to lie anywhere between the step positions for each of the two separate phases.
Closed-loop current control is needed to prevent the current from changing as a result of temperature changes in the windings, or variations in the supply voltage; and if it is necessary to ensure that the holding torque stays constant for each mini-step both currents must be changed according to a prescribed algorithm.
Despite the difficulties referred to above, mini-stepping is used extensively, especially in photographic and printing applications where a high resolution is needed.
Schemes involving between 3 and 10 mini-steps for a 1.8o step motor are numerous, and there are instances where up to 100 mini-steps (20,000 mini-steps/rev) have been successfully achieved.
So far, we have concentrated on those aspects of behaviour, which depend only on the motor itself, i.e. the static performance. The shape of the static torque curve, the holding torque and the slope of the torque curve about the step position have all been shown to be important pointers to the way the motor can be expected to perform.
All of these characteristics depend on the current(s) in the windings, however, and when the motor is running the instantaneous currents will depend on the type of drive circuit employed.
Steady State Characteristics of Stepping Motors
In this section, we will look at how the motor would perform if it were supplied by an ideal drive circuit, which turns out to be one that is capable of supplying rectangular pulses of current to each winding when required, and regardless of the stepping rate.
Because of the inductance of the windings, no real drive circuit will be able to achieve this, but the most sophisticated (and expensive) ones achieve near-ideal operation up to very high stepping rates.
Requirements of Drive
The basic function of the complete drive is to convert the step command input signals into appropriate patterns of currents in the motor windings. This is achieved in two distinct stages, as shown in Figure 4, which relates to a 3-phase motor.
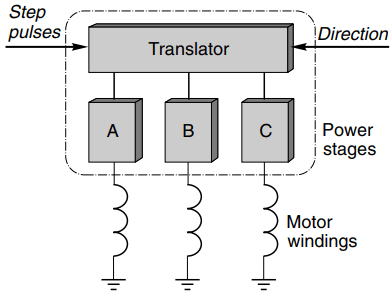

The ‘translator’ stage converts the incoming train of step command pulses into a sequence of on/off commands to each of the three power stages. In the one-phase-on mode, for example, the first step command pulse will be routed to turn on phase A, the second will turn on phase B and so on.
In a very simple drive, the translator will probably provide for only one mode of operation (e.g. one-phase-on), but most commercial drives provide the option of one-phase-on, two-phase-on and half stepping.
Single-chip ICs with these three operating modes and with both three-phase and four-phase outputs are readily available. The power stages (one per phase) supply the current to the windings.
An enormous diversity of types are in use, ranging from simple ones with one switching transistor per phase, to elaborate chopper-type circuits with four transistors per phase.
At this point, however, it is helpful to list the functions required of the ‘ideal’ power stage. These are
- firstly that when the translator calls for a phase to be energised, the full (rated) current should be established immediately;
- secondly, the current should be maintained constant (at its rated value) for the duration of the ‘on’ period
- and finally, when the translator calls for the current to be turned off, it should be reduced to zero immediately.
The ideal current waveforms for continuous stepping with one-phase-on operation are shown in the lower part of Figure 4. The currents have a square profile because this leads to the optimum value of running torque from the motor.
But because of the inductance of the windings, no real drive will achieve the ideal current waveforms, though many drives come close to the ideal, even at quite high stepping rates. Drives which produce such rectangular current waveforms are (not surprisingly) called constant-current drives.
We now look at the running torque produced by a motor when operated from an ideal constant current drive. This will act as a yardstick for assessing the performance of other drives, all of which will be seen to have inferior performance.
Pull-out Torque under Constant-current Conditions
If the phase currents are taken to be ideal, i.e. they are switched-on and switched-off instantaneously, and remain at their full-rated value during each ‘on’ period, we can picture the axis of the magnetic field to be advancing around the machine in a series of steps, the rotor being urged to follow it by the reluctance torque.
If we assume that the inertia is high enough for fluctuations in rotor velocity to be very small, the rotor will be rotating at a constant rate, which corresponds exactly to the stepping rate.
Now if we consider a situation where the position of the rotor axis is, on average, lagging behind the advancing field axis, it should be clear that, on average, the rotor will experience a driving torque. The more it lags behind, the higher will be the average forward torque acting on it, but only up to a point.
We already know that if the rotor axis is displaced too far from the field axis, the torque will begin to diminish, and eventually reverse, so we conclude that although more torque will be developed by increasing the rotor lag angle, there will be a limit to how far this can be taken.
Turning now to a quantitative examination of the torque on the rotor, we will make use of the static torque–displacement curves discussed earlier, and look at what happens when the load on the shaft is varied, the stepping rate being kept constant.
Clockwise rotation will be studied, so the phases will be energised in the sequence ABC. The instantaneous torque on the rotor can be arrived at by recognising
- that the rotor speed is constant, and it covers one-step angle (30o) between step command pulses, and
- the rotor will be ‘acted on’ sequentially by each of the set of torque curves.
When the load torque is zero, the net torque developed by the rotor must be zero (apart from a very small torque required to overcome friction). This condition is shown in Figure 5(a).
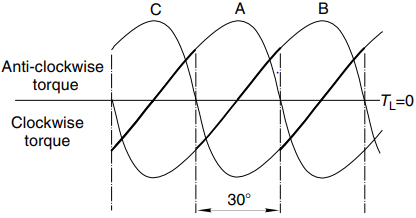
The instantaneous torque is shown by the thick line, and it is clear that each phase in turn exerts first a clockwise torque, then an anticlockwise torque while the rotor angle turns through 30o. The average torque is zero, the same as the load torque, because the average rotor lag angle is zero.
When the load torque on the shaft is increased, the immediate effect is to cause the rotor to fall back in relation to the field. This causes the clockwise torque to increase, and the anticlockwise torque to decrease.

Equilibrium is reached when the lag angle has increased sufficiently for the motor torque to equal the load torque. The torque developed at an intermediate load condition like this is shown by the thick line in Figure 5(b).
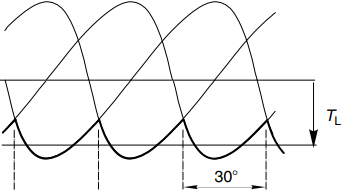
The highest average torque that can possibly be developed is shown by the thick line in Figure 5(c): if the load torque exceeds this value (which is known as the pull-out torque) the motor loses synchronism and stalls, and the vital one-to-one correspondences between pulses and steps are lost.
Since we have assumed an ideal constant-current drive, the pull-out torque will be independent of the stepping rate, and the pull-out torque– speed curve under ideal conditions is therefore as shown in Figure 6.
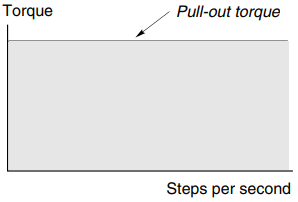
The shaded area represents the permissible operating region: at any particular speed (stepping rate) the load torque can have any value up to the pull-out torque, and the motor will continue to run at the same speed. But if the load torque exceeds the pull-out torque, the motor will suddenly pull out of synchronism and stall.
As mentioned earlier, no real drive will be able to provide the ideal current waveforms, so we now turn to look briefly at the types of drives in common use, and at their pull-out torque–speed characteristics.