The self-generating thermocouple is one of the more widely used (but most misunderstood) sensors for routine and specialty thermometry because of its versatility, simplicity, ease of use, and low cost. It employs the Seebeck thermoelectric effect that, unlike the related Peltier and Thomson thermoelectric effects, alone converts heat to emf.
The physical cause of thermoelectric phenomena is complex in detail as applied to real materials. It is explained by modern theory confirmed by experiment. Seebeck properties of many materials can be predicted. For reliable thermometry, it is not essential to understand the underlying physics.
For application, it is sufficient to understand a few very simple but general thermoelectric principles that are fully consistent with physical theory and experiment.
Absolute Seebeck Effect
The absolute Seebeck thermoelectric effect, the occurrence of a net emf between two points of a conductor because their temperatures are different, results from the temperature-dependent distribution of charge carriers.
Seebeck emf occurs without dissimilar materials. It is not a junction phenomenon, nor is it the same as Volta’s contact potential. It is related to but is not the result of the associated Peltier or Thomson thermoelectric effects that are insignificant in thermocouple thermometry.
To practice and to fully apply the simple principles, it also is not necessary to understand calculus. Nevertheless, the application principles are developed as follows. The absolute Seebeck coefficient expresses the measurement sensitivity (volts per unit of temperature) of the Seebeck effect.
It is defined over any thermoelectrically homogeneous region, x, along a slender conducting material
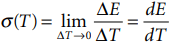
Where, ∆E is the net Seebeck emf across the homogeneous segment.
∆T is the temperature difference between ends of that region.
The Seebeck coefficient, σ(T), is a physical transport property of all individual electrically conducting materials.
From Equation 1.1, the net emf is
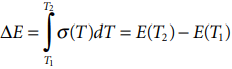
Where ∆E is simply the difference of emf between any pair of points of a homogeneous segment, separated by any distance, x, between which the temperature difference is ∆T = (T2 − T1).
Note that the net Seebeck emf for such a homogeneous segment depends only on the temperatures at the two segment endpoints and not on the local values of temperature or temperature gradients, dT/dx, between them, nor on the distance between those two points.
An overall inhomogeneous thermoelement is composed of locally homogeneous segments. Nevertheless, for general thermometry, each entire thermoelement must be homogeneous so that only endpoint temperatures, not temperature distribution, matter.
The Seebeck coefficient σ(T) is a nonlinear function of temperature. For meaningful thermometry, it must be a function of temperature alone, independent of time or location along a thermoelement.
Although it is vulnerable to environmental effects, for accurate thermometry, it must not depend on such environmental variables as strain, pressure, or magnetic field during measurement.
The coefficient, σM(T), is an absolute Seebeck coefficient for a particular material, M. The corresponding source voltage within a single material is an absolute Seebeck emf, EM(T).
The absolute Seebeck emf does physically exist, but it is directly observable over only a limited cryogenic temperature range near 0 K where it can be related to a superconductive thermoelement reference that has, over the range, a negligible Seebeck coefficient. The absolute Seebeck coefficient at higher temperatures is usually determined indirectly.
The Thomson coefficient, τ(T), of the individual material is measured. The Seebeck coefficient is determined from a thermodynamic Kelvin relationship as follows:
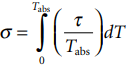
Relative Seebeck Characteristics
The difference between the Seebeck emfs of two thermoelements, of materials A and B, with one end of each at temperature, Tm, and the other ends both at temperature, Tr is their relative Seebeck emf, EAB(T), expressed as
EAB(Tm,Tr) = EA(Tm,Tr) – EB(Tm,Tr) ………(Equation 1.4)
The corresponding relative Seebeck coefficient at Tm for a pair is
σAB(Tm) = σA(Tm) – σB(Tm) ……(Equation 1.5)
Note that these relationships apply whether or not the thermoelements are joined at a junction.
The thermally paired segments often are remote in a circuit. It is this relative Seebeck coefficient that has been called by the anachronistic term “thermopower.” (Thermoelectric power appropriately refers to electrical power generated using the Seebeck effect.) It is these relative voltage or coefficient values that are directly observable and are used in thermoelectric thermometry.
These are the values that are presented in the tables of thermocouple emf vs. measuring junction temperature, Tm, referred to a designated reference temperature, Tr. Standardized thermocouple characteristics, by convention, are referenced to Tr = 0 °C.
Terminology
Thermocouple: Any pair of electrically conducting thermoelectrically different material legs in Ohmic contact at a junction is a thermocouple. The individual legs are thermoelements. Thermoelements can be solid or liquid, normal-, semi-, or superconductors. Seebeck emf occurs only in non-isothermal thermoelements, not junctions.
Junction: Any electrically conducting interface between dissimilar thermoelements is a thermoelectric junction. Junctions provide electrical connectivity. Junctions contribute no Seebeck emf. Therefore, calibration is of thermoelement segments, not of junctions. Junctions define thermoelement endpoint temperatures and thereby “sense” temperature. Electrical connections between like thermocouple legs are splices, not junctions.
Sensitivity: The sensitivity, emf versus temperature, of each individual leg material is expressed by an absolute Seebeck coefficient. Net emf between thermally paired dissimilar thermoelements is described by the more familiar relative Seebeck coefficient.
Thermocouple states: Segments of thermoelements are isothermal where they are at uniform temperature. Multiple junctions are isothermal for thermometry while they are acceptably near the same temperature.
A thermoelement is thermoelectrically homogeneous along any segment where its Seebeck coefficient is uniform. It is inhomogeneous for thermometry where spatial nonuniformity of the absolute Seebeck coefficient could significantly degrade thermometry.
Significant hidden inhomogeneity resulting from use is a commonplace and usually unrecognized problem in practical thermometry and calibration.
Related Posts
- Thermocouple Working Principle
- Absolute Seebeck Effect
- Basic Thermocouple Circuits
- Extensions of Thermocouple
- Functional Model of Thermoelectric Circuits
- T/X Sketch of Dual-Reference Junction Circuit
- Applications of Functional Model
- Characteristics of Thermocouples
- Thermocouple Hardware
- Thermocouple Junction Styles
- Active Tests of Thermocouple
- Calibration of Thermocouples
- Thermocouple Thermometry Practice
- Distinctive Thermocouple Noise Problems